Question

Q varies inversely as the square of p, and Q = 36 when p = 7. Find Q when p = 6.

A. Q = 176
B. Q = 6
C. Q = 49
D. Q = 42

Please no guesses and I'd like to see an explanation if possible because I'm reviewing for a final.

Answer 1

For this case we have that by definition, a direct variation is represented as:

`y = kx`

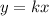
While an inverse variation is represented as:

`y = \frac {k} {x}`

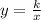
If we have that Q varies inversely as the square of p means that:

`Q = \frac {k} {p ^ 2}`

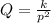
Substituting the values and clearing the proportionality constant we have:

`36 = \frac {k} {7 ^ 2}\\36 = \frac {k} {49}\\k = 36 * 49\\k = 1764`

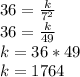
Now we must find the value of Q when
`p = 6`:

`Q = \frac {k} {6 ^ 2}\\Q = \frac {1764} {36}\\Q = 49`

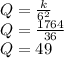
Answer:

Option C