Answer:
Given : Sample mean = x = 5.25
Standard deviation = 0.52
To Find : Use a 0.01 significance level and the given calculator display to test the claim that the sample is from a population with a mean less than 5.4
Solution:
Sample size = n = 58
Hypothesis :
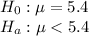
Test statistic:
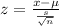
x = 5.25
μ = 5.4
s = 0.52
n = 58
Substitute the values :
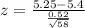
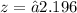
Refer the p value using z table
P-value = P(z ≤ -2.196) = 0.0143
As P-value > 0.01,
So, we accept the null hypothesis
Conclusion: There is significant statistical evidence to conclude that the claim that the sample is from a population with a mean less than 5.4 is False at alpha = 0.01, the level of significance.