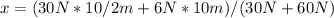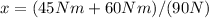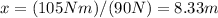Answer:
The beam must be picked up at 8.33m respect to the opposite corner from the weight (to 1.66 m from the weight).
Step-by-step explanation:
As we want the beam to be balanced, we will define the moment of the beam and the weight respect to an arbitrary point x from the opposite corner to the weight. The beam weight will be placed at the half of the beam:
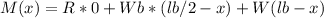
(See the ilustration below to guide)
Where Wb and lb are the weight and the length of the beam.
Note that the zero distance from the picked-up force is due the force is located at the arbitrary point so no moment is doing.
Now due the beam has to be balanced the total moment must be zero:

Then is possible to find the x position point by solving the equation above:
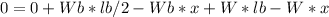
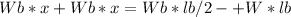


For the respecting values: