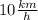Question

A motorboat travels 92 km in 2 hours going upstream. It travels 132 km going downstream in the same amount of time. What is the rate of the boat in still water and what is the rate of the current?

Answer 1

The speed on boat in still water is
`56 (km)/(h)` and the rate of the current is
`10 (km)/(h)`

Step-by-step explanation:

Since speed ,
`v= (Distance\, traveled(D))/(Time\, taken(t))`

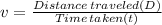
Therefore speed of motor boat while traveling upstream is

`v_(upstream)=(92)/(2)(km)/(h)=46(km)/(h)`

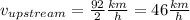
and speed of motor boat while traveling downstream is

`v_(downstream)=(132)/(2)(km)/(h)=66(km)/(h)`

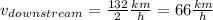
Let speed of boat in still water be
`v_b` and rate of current be
`v_w`

Therefore
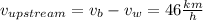`v_(upstream)=v_b-v_w=46(km)/(h)` ----(A)

and
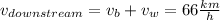`v_(downstream)=v_b+v_w=66(km)/(h)` ------(B)

Adding equation (A) and (B) we get

`2v_b= (46+66) (km)/(h)=112 (km)/(h)`

=>
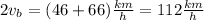
`v_b= 56 (km)/(h)` ------(C)

Substituting the value of
`v_b` in equation (A) we get

`v_w= 10 (km)/(h)`

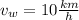
Thus the speed on boat in still water is
`56 (km)/(h)` and the rate of the current is
`10 (km)/(h)`