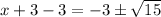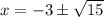Answer:
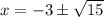
Explanation:
We have the following equation
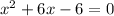
To use the method of completing squares you must take the coefficient of x and divide it by 2 and square the result.
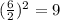
Now add 9 on both sides of equality
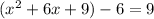
Factor the term in parentheses
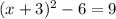
Add 6 on both sides of the equation
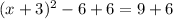

Take square root on both sides of the equation

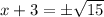
Subtract 3 from both sides of the equation.