Question

Number 28 is the only question I need please help, with steps

Answer 1

f (gx) = 1/ -2(1/x^2+6x+10) + 9

Explanation:

f (gx) = 1/ -2(1/x^2+6x+10) + 9

Answer 2

The domain is all real numbers where

`(f \circ g)(x)=(x^2+6x+10)/(9x^2+54x+88)`

Explanation:

`(f \circ g)(x)=f(g(x))`

So g(x) must exist before plugging it into f(x).

Let's find where g(x) doesn't exist.

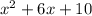`x^2+6x+10` is a quadratic expression.

`b^2-4ac` is the discriminant and will tell us if
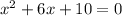`x^2+6x+10=0` will have any solutions. I'm trying to solve this equation because I want to figure out what to exclude from the domain. Depending on what
`b^2-4ac` we may not have to go full quadratic formula on this problem.

`b^2-4ac=(6)^2-4(1)(10)=36-40=-4`.

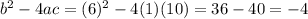Since the discriminant is negative, then there are no real numbers that will make the denominator 0 here. So we have no real domain restrictions on g.

Let's go ahead and plug g into f.

`f(g(x))`

`f((1)/(x^2+6x+10))`

I replaced g(x) with (1/(x^2+6x+10)).

`(1)/(-2((1)/(x^2+6x+10))+9)`

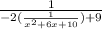
I replaced old input,x, in f with new input (1/(x^2+6x+10)).

Let's do some simplification now.

We do not like the mini-fraction inside the bigger fraction so we are going to multiply by any denominators contained within the mini-fractions.

I'm multiplying top and bottom by (x^2+6x+10).

`(1)/(-2((1)/(x^2+6x+10))+9) \cdot ((x^2+6x+10))/((x^2+6x+10))`

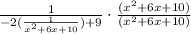
Using distributive property:

`(1(x^2+6x+10))/(-2((1)/(x^2+6x+10))\cdot(x^2+6x+10)+9(x^2+6x+10))`

We are going to distribute a little more:

`(x^2+6x+10)/(-2+9x^2+54x+90)`

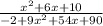
Combine like terms on the bottom there (-2 and 90):

`(x^2+6x+10)/(9x^2+54x+88)`

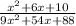
Now we can see if we have any domain restrictions here:

`b^2-4ac=(54)^2-4(9)(88)=-252`

So again the bottom will never be zero because
`9x^2+54x+88=0` doesn't have any real solutions. We know this because the discriminant is negative.

The domain is all real numbers where

`(f \circ g)(x)=(x^2+6x+10)/(9x^2+54x+88)`