Answer:
New houses build =200
Profit =$13,860,000
Step-by-step explanation:
In this particular question there are 2 scenarios for demand function,
i.e. (a.) 60 percent chance of low demand,
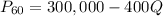
(b.) 40 percent chance of high demand,

∴ Expected demand function = 60%×(300000-400Q) + 40%×(500000-275Q)
= 380,000-350Q
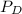 =380000-350Q
=380000-350Q
Revenue = (380000 - 350Q)×Q = 380000Q - 350
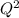
Here, the no. of new homes build will depend on maximizing profit
∵ Profit = Revenue - Cost
π = (380000 - 350Q)×Q - (140000+240000Q)
π = 380000Q - 350
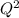 - (140000+240000Q)
- (140000+240000Q)
In order to maximize profit , we will
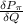 = 0
= 0
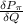 = 380000-350*2*Q-240000
= 380000-350*2*Q-240000
∴ 380000-350×2×(Q-240000) =0
Q=200
So number of houses that they should build =200
π = 380000Q - 350
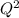 - (140000+240000Q)
- (140000+240000Q)
π = 380000-(350×
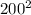 ) - (140000+(240000*200))
) - (140000+(240000*200))
π =$13,860,000
New houses to be build =200
Profit =$13,860,000