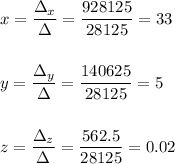Question

Gold used to make jewerly is often a blend of​ gold, silver, and copper. Consider three alloys of these metals. The first alloy is​ 75% gold,​ 5% silver, and​ 20% copper. The second alloy is​ 75% gold,​ 12.5% silver, and​ 12.5% copper. The third alloy is​ 37.5% gold and​ 62.5% silver. If 100 g of the first alloy costs ​$2500.40​, 100 g of the second alloy costsnbsp $ 2537.75​, and 100 g of the third alloy costs $ 1550.00​, how much does each metal​ cost?

Answer 1

Gold - $33, Silver - $5, Copper - $0.02

Explanation:

Let $x be the price of one gram of gold, $y - price of 1 g of silver and $z - price of 1 g of copper.

1. The first alloy is​ 75% gold,​ 5% silver, and​ 20% copper, so in 100 g there are 75 g of gold, 5 g of silver and 20 g of copper. If 100 g of the first alloy costs ​$2500.40​, then

75x+5y+20z=2500.40

2. The second alloy is​ 75% gold,​ 12.5% silver, and​ 12.5% copper, so in 100 g there are 75 g of gold, 12.5 g of silver and 12.5 g of copper. If 100 g of the first alloy costs ​$2537.75​, then

75x+12.5y+12.5z=2537.75

3. The third alloy is​ 37.5% gold and​ 62.5% silver, so in 100 g there are 37.5 g of gold and 62.5 g of silver . If 100 g of the first alloy costs ​$1550.00​, then

37.5x+62.5y=1550.00

Solve the system of three equations:

`\left\{\begin{array}{l}75x+5y+20z=2500.40\\75x+12.5y+12.5z=2537.75\\37.5x+62.5y=1550.00\end{array}\right.`

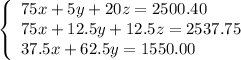
Find all determinants

`\Delta=\|\left[\begin{array}{ccc}75&5&20\\75&12.5&12.5\\37.5&62.5&0\end{array}\right] \|=28125\\ \\</p><p>\Delta_x=\|\left[\begin{array}{ccc}2500.40&5&20\\2537.75&12.5&12.5\\1550.00&62.5&0\end{array}\right] \|=928125\\ \\</p><p>\Delta_y=\|\left[\begin{array}{ccc}75&2500.40&20\\75&2537.75&12.5\\37.5&1550&0\end{array}\right] \|=140625\\ \\</p><p>\Delta_z=\|\left[\begin{array}{ccc}75&5&2500.40\\75&12.5&2537.75\\37.5&62.5&1550\end{array}\right] \|=562.5\\ \\`

So,

`x=(\Delta_x)/(\Delta)=(928125)/(28125)=33\\ \\\\y=(\Delta_y)/(\Delta)=(140625)/(28125)=5\\ \\\\z=(\Delta_z)/(\Delta)=(562.5)/(28125)=0.02\\ \\`