Answer:
Domain: Set of All Real Numbers. (-∞, ∞)
Range: (0, ∞)
Explanation:
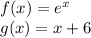
We need to evaluate (gof)(x). (gof)(x) means the composition of function g(x) and f(x). In order to find (gof)(x) replace every occurrence of x in g(x) with the expression of f(x) as shown below:
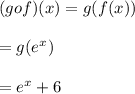
We have to find the domain and range of this composite function.
Domain:
The expression
 is defined for all Real Numbers. It never gets undefined for any value of x. Hence the Domain is set of All Real Numbers.
is defined for all Real Numbers. It never gets undefined for any value of x. Hence the Domain is set of All Real Numbers.
Range:
The range of the parent exponential function
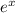 is from 0 to positive infinity. The expression
is from 0 to positive infinity. The expression
 can be related to
can be related to
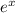 as a vertical shifted function by 6 units upward. As a result the range will also be shifted up. So the range of the composite function will be from 6 to positive infinity. In interval notation this would be expressed as: (0, ∞)
as a vertical shifted function by 6 units upward. As a result the range will also be shifted up. So the range of the composite function will be from 6 to positive infinity. In interval notation this would be expressed as: (0, ∞)