Answer:
The correct option is 1.
Explanation:
It is given that a system of inequalities can be used to determine the depth of a toy, in meters, in a pool depending on the time, in seconds, since it was dropped.
Let y be the depth of a toy and x is time, in seconds.
In the given graph a solid horizontal line passes through the point (0,-1) and shaded region is above the line. So, the inequality of red line is
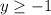
The depth of a toy can be less than -1. It means the pool is 1 meter deep.
The blue line is a dashed line which passes through (0,0) and (2,-1).
So the slope of line is

The equation of blue line is

where, m is slope and b is y-intercept.
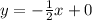

The shaded region is below the line so the required inequality is

it means the toy sinks at a rate of less than 1/2 meter per second.
Therefore the correct option is 1.