Question

The two shafts of a Hooke’s coupling have their axes inclined at 20°.The shaft A revolves at a uniform speed of 1000 rpm. The shaft B carries a flywheel of mass 30 kg. If the radius of gyration of the flywheel is 100 mm, find the maximum torque in shaft B.

Answer 1

33.429 N-m

Step-by-step explanation:

Given :

Inclination angle of two shaft, α = 20°

Speed of shaft A,
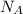`N_(A)` = 1000 rpm

Mass of flywheel, m = 30 kg

Radius of Gyration, k =100 mm

= 0.1 m

Now we know that for maximum velocity,

`(N_(B))/(N_(A)) = (cos\alpha )/(1 - sin^(2)\alpha )`

`(N_(B))/(1000) = (cos20)/(1 - sin^(2)20 )`

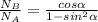
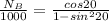
`N_(B)` = 1064.1 rpm

Now we know

Mass of flywheel, m = 30 kg

Radius of Gyration, k =100 mm

= 0.1 m

Therefore moment of inertia of flywheel, I = m.
`k^(2)`

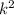
=30 X
`0.1^(2)`

= 0.3 kg-
`m^(2)`

Now torque on the output shaft

T₂ = I x ω

= 0.3 X 1064.2 rpm

=
`0.3* (2\pi * 1064.1)/(60)`

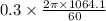
= 33.429 N-m

Torque on the Shaft B is 33.429 N-m