Answer with Step-by-step explanation:
Let A element of
 (R) be a diagonalizable matrix with
(R) be a diagonalizable matrix with
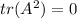 given
given
We have to prove that A is the zero matrix
Le A=
![\left[\begin{array} {ccc}a_(11)&0&0\\0&a_(22)&0\\0&0&a_(33)\end{array}\right]](https://img.qammunity.org/2020/formulas/mathematics/college/xeer9umuxxvcq4bil6137snewcipn21ik3.png) be any matrix of order
be any matrix of order
Trace : Trace is defined as the sum of diagonal elements of a matrix.
![A* A=A^2=\left[\begin{array}{ccc}a_(11)&0&0\\0&a_(22)&0\\0&0&a_(33)\end{array}\right]* \left[\begin{array}{ccc}a_(11)&0&0\\0&a_(22)&0\\0&0&a_(33)\end{array}\right]](https://img.qammunity.org/2020/formulas/mathematics/college/habvpotyem65wmiwmwbsspt4p2zakf0b24.png)
![A^2=\left[\begin{array} {ccc}a^2_(11)&0&0\\0&a^2_(22)&0\\0&0&a^2_(33)\end{array}\right]](https://img.qammunity.org/2020/formulas/mathematics/college/cg1bwhruz031efs7a8sb1s5ri2n0dzimql.png)
Therefore,
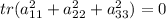
We know that square of an positive or negative element is positive
Therefore, it is necessary that
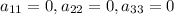 when tr(
when tr(
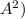 =0
=0
When all elements of matrix are zero then the matrix should be zero matrix.
Hence, A is a zero matrix .