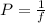Question

A far sighted person can not see clearly objects that are closer to his eyes than 60.0 cm. Which one of the following combinations represents the correct focal length and the refractive power of the contact lenses that will enable him to see the objects at a distance of 25.0 cm from his eyes?

A) -42.9 cm, +2.33 diopters

B) -42.9 cm, -2.33 diopters

C) +60 cm, +42.9 diopters

D) +42.9 cm, +2.33 diopters

E) +42.9 cm, -2.33 diopters

Answer 1

`f = +42.9 cm`

`P =+2.33Dioptre`

Step-by-step explanation:

As we know that Far sighted person has near point shifted to 60 cm distance

so he is able to see the object 60 cm

and the person want to see the objects at distance 25 cm

so here the image distance from lens is 60 cm and the object distance from lens is 25 cm

now from lens formula we have

`(1)/(d_i) + (1)/(d_0) = (1)/(f)`

`-(1)/(60) + (1)/(25) = (1)/(f)`

`f = +42.9 cm`

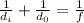
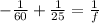
Now we know that power of lens is given as

`P = (1)/(f)`

`P = (1)/(0.429) = +2.33Dioptre`