Question

Y = x2 − 6x + 8

2y + x = 4
The pair of points representing the solution set of this system of equations is

Answer 1

The two intersections are (3/2 , 5/4) and (4,0).

I put two ways to do it. You can pick your favorite of these are try another route if you like.

Explanation:

The system is:

`y=x^2-6x+8`

`2y+x=4`.

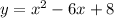
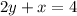I don't know how good at factoring you are but the top equation consists of polynomial expression that has a factor of (x-4). I see that if I solve 2y+x=4 for 2y I get 2y=-x+4 which is the opposite of (x-4) so -2y=x-4.

So anyways, factoring x^2-6x+8=(x-4)(x-2) because -4+(-2)=-6 while -4(-2)=8.

This is the system I'm looking at right now:

`y=(x-4)(x-2)`

`-2y=x-4`

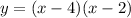
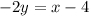
I'm going to put -2y in for (x-4) in the first equation:

`y=-2y(x-2)`

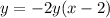
So one solution will occur when y is 0.

Now assume y is not 0 and divide both sides by y:

`1=-2(x-2)`

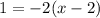
Distribute:

`1=-2x+4`

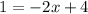
Subtract 4 on both sides:

`-3=-2x`

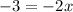
Divide both sides by -2:

`(-3)/(-2)=x`

`(3)/(2)=x`

`x=(3)/(2)`

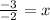
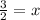
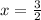
Now let's go back to one of the original equations:

2y=-x+4

Divide both sides by 2:

`y=(-x+4)/(2)`

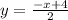
Plug in 3/2 for x:

`y=((-3)/(2)+4)/(2)`

Multiply top and bottom by 2:

`y=(-3+8)/(4)`

`y=(5)/(4)`

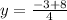
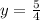
So one solution is at (3/2 , 5/4).

The other solution happened at y=0:

2y=-x+4

Plug in 0 for y:

2(0)=-x+4

0=-x+4

Add x on both sides:

x=4

So the other point of intersection is (4,0).

-------------------------------------------------------

The two intersections are (3/2 , 5/4) and (4,0).

Now if you don't like that way:

`y=x^2-6x+8`

`2y+x=4`

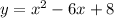
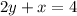
Replace y in bottom equation with (x^2-6x+8):

`2(x^2-6x+8)+x=4`

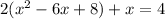
Distribute:

`2x^2-12x+16+x=4`

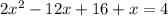
Subtract 4 on both sides:

`2x^2-12x+16+x-4=0`

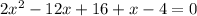
Combine like terms:

`2x^2-11x+12=0`

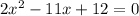
Compare this to
`ax^2+bx+c=0`

`a=2`

`b=-11`

`c=12`

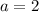
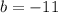
The quadratic formula is

`x=(-b \pm √(b^2-4ac))/(2a)`

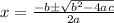
{Plug in our numbers:

`x=(11 \pm √((-11)^2-4(2)(12)))/(2(2))`

`x=(11 \pm √(121-96))/(4)`

`x=(11 \pm √(25))/(4)`

`x=(11 \pm 5)/(4)`

`x=(11+5)/(4) \text{ or } (11-5)/(4)`

`x=(16)/(4) \text{ or } (6)/(4)`

`x=4 \text{ or } (3)/(2)`

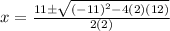
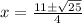
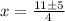
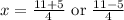
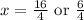
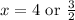
Using 2y+x=4 let's find the correspond y-coordinates.

If x=4:

2y+4=4

Subtract 4 on both sides:

2y=0

Divide both sides by 2:

y=0

So we have (4,0) is a point of intersection.

If x=3/2

2y+(3/2)=4

Subtract (3/2) on both sides:

2y=4-(3/2)

2y=5/2

Divide 2 on both sides:

y=5/4

The other intersection is (3/2 , 5/4).