Answer:
0.8413 or 84.13%
Explanation:
Given : The mean is 72 inches and the standard deviation is 15 inches
To Find : What is the probability that in a randomly selected year, the snowfall was less than 87 inches
Solution:
Mean =
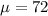
Standard deviation =
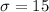
Formula :

We are supposed to find the probability that in a randomly selected year, the snowfall was less than 87 inches
So, x = 87
Substitute the values in the formula

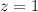
Now to find P(z<87) refer the z table
P(Z<87)=0.8413 = 84.13%
So, the probability that in a randomly selected year, the snowfall was less than 87 inches if the mean is 72 inches and the standard deviation is 15 inches is 0.8413 or 84.13%