Answer:
(a) No. (b)Yes. (c)Yes. (d)Yes.
Explanation:
(a) If
 is an homomorphism, then it must hold
is an homomorphism, then it must hold
that
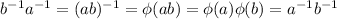 ,
,
but the last statement is true if and only if G is abelian.
(b) Since G is abelian, it holds that
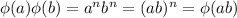
which tells us that
 is a homorphism. The kernel of
is a homorphism. The kernel of

is the set of elements g in G such that
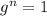 . However,
. However,
 is not necessarily 1-1 or onto, if
is not necessarily 1-1 or onto, if
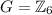 and
and
n=3, we have
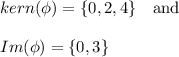
(c) If
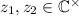 remeber that
remeber that
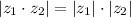 , which tells us that
, which tells us that
 is a
is a
homomorphism. In this case
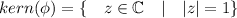 , if we write a
, if we write a
complex number as
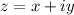 , then
, then
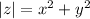 , which tells
, which tells
us that
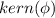 is the unit circle. Moreover, since
is the unit circle. Moreover, since
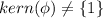 the mapping is not 1-1, also if we take a negative
the mapping is not 1-1, also if we take a negative
real number, it is not in the image of
 , which tells us that
, which tells us that
 is not surjective.
is not surjective.
(d) Remember that
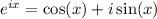 , using this, it holds that
, using this, it holds that
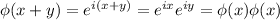
which tells us that
 is a homomorphism. By computing we see
is a homomorphism. By computing we see
that
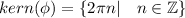 and
and
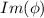 is the unit circle, hence
is the unit circle, hence
 is neither injective nor
is neither injective nor
surjective.