Answer:
Option B

Explanation:
we know that
The critical point of a function are the points on the graph of a function where the derivative is zero or the derivative does not exist.
we have
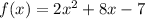
step 1
Take the derivative of the function
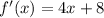
step 2
Set that derivative equal to 0 and solve for x. Each x value you find is known as a critical number
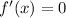
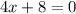
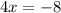

Alternative Method
The critical point of the quadratic equation is the vertex, because the function changes from decreasing to increasing at that point (In this problem the vertex is a minimum)
we have
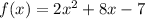
Convert into vertex form
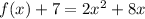
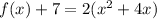
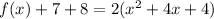

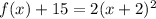
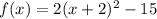
the vertex is the point (-2,-15)
therefore
The x-coordinate of the critical point is x=-2