Question

A researcher is interested in consumer preferences among three brands of yogurt. A sample of 90 people is obtained,

and each individual is asked to taste each brand and then select his/her favorite. The resulting frequency data are as

follows:

Brand A

Brand B Brand C

37

21

32

Do the data indicate any significant preferences among the three brands? Test at the .05 level of significance.

State the null hypothesis (1 point), the expected frequency for each category (3 points), the value of chi-

square (4 points), the df (1 points), the critical value of chi-square (2 points), and the decision regarding

significance (1 points).

Answer 1

Explanation:

From the given information:

The null hypothesis: all three brands are equally likely to their expected value

The alternative hypothesis: preferences exist among the three brands i.e. at least one is different from the expected value.

The expected frequency =
`f_e`

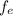
The observed frequency =
`f_o`

`f_e`
`f_o`
`(f_o- f_e)^2`
`((f_o - f_e)^2)/(f_e)`

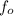
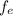
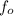
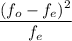
37 30 49.00 1.633

21 30 81.00 2.700

32 30 4.00 0.133

`X^2 = \sum((f_o-f_e)^2)/(f_e) = 4.47`

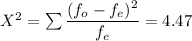
Degree of freedom =
`(n-1)`

= 3 -1

= 2

At ∝ = 0.05 and df = 2

The critical value
`X^2_(0.05, 2)= 5.99`

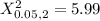
Decision rule:

Since the calculated chi-square is less than the critical value, we do not reject the null hypothesis.

Thus, the null is retained with a calculated chi-square value of 4.47 and a critical chi-square value of 5.99.