Question

What is the most precise name for quadrilateral ABCD with vertices A(−5,7), B(6,−3), C(10,2), and D(−1,12)?

Answer 1

Answer:Answer:

the figure is parallelogram

Explanation:

enter image source here

As The mutually edges are parallel each other and equal,

the name of figure is parallelogram .

Explanation:

Answer 2

Answer with explanation:

The vertices of Quadrilateral ABCD are ,A(−5,7), B(6,−3), C(10,2), and D(−1,12).

Distance formula between two points (a,b) and (c,d), is given by

`=\sqrt{(a-c)^2+(b-d)^2`

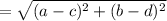
`AB=√([-5-6]^2+[7-(-3)]^2)\\\\AB=√(121+100)\\\\AB=√(221)\\\\BC=√([10-6]^2+(2+3)^2)\\\\BC=√(16+25)\\\\BC=√(41)\\\\CD=√([10+1]^2+[2-12]^2)\\\\CD=√(121+100)\\\\CD=√(221)\\\\DA=√([-1+5]^2+[12-7]^2)\\\\DA=√(16+25)\\\\DA=√(41)\\\\AC=√([10+5]^2+[2-7]^2)\\\\AC=√(225+25)\\\\AC=√(250)\\\\BD=√([6+1]^2+[-3-12]^2])\\\\BD=√(49+225)\\\\BD=√(274)`

Opposite side of Quadrilateral[AB=CD, AD=BC] is equal, but Diagonals are not equal.

So, it is a Parallelogram.