Answer:
The only integer solution is n1=2
Explanation:
Let's find the answer by using the following equation applied to quadratic polynomials with the form
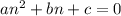 :
:
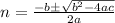
For our case
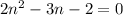 , a=2, b=-3, and c=-2, so:
, a=2, b=-3, and c=-2, so:

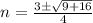
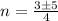

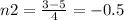
In conclusion, although there are 2 roots (n1, n2), only one of them is an integer, which is n1=2.