Answer:
C. x =3
Explanation:
Extraneous solution is that root of a transformed equation that doesn't satisfy the equation in it's original form because it was excluded from the domain of the original equation.
Let's solve the equation first
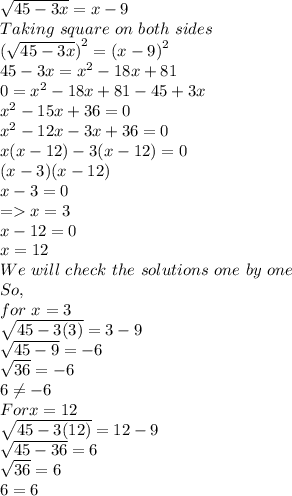
Hence, we can conclude that x=3 is an extraneous solution of the equation ..