Question

Rectangle with a side length of 11" and a diagonal of 14" what is the perimeter

Answer 1

10sqrt3+22

Explanation:

Ok, let us imagine it as a sort of rectangle split upon its diagonal.

Using that, we can Pythag it out,

11^2+b^2=14^2

121+b^2=196

b^2=75

b=sqrt75

b=5sqrt3

Ok, using this info, we find the perimeter,

5sqrt3+5sqrt3+11+11

10sqrt3+22

The answer is 10sqrt3+22

Answer 2

Hello!

The answer is:

The perimeter of the rectangle is equal to 39.32".

`Perimeter=39.32in`

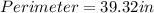
Why?

Since we are working with a rectangle, we can use the Pythagorean theorem to find the missing side of the rectangle and calculate its perimeter. We must remember that we can divide a rectangle into two equal right triangles.

According to the Pythagorean Theorem, we have:

`a^(2)=b^(2)+c^(2)`

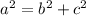
Where:

a, represents the hypotenuse of the triangle which is equal to the diagonal of the given rectangle (14")

b and c are the other sides of the triangle.

Now, let be "a" 14" and "b" 11"

So, solving we have:

`a^(2)=b^(2)+c^(2)`

`14^(2)=11^(2)+c^(2)`

`14^(2)-11^(2)=c^(2)`

`14^(2)-11^(2)=c^(2)\\\\c=\sqrt{14^(2) -11^(2) }=√(196-121)=√(75)=8.66in`

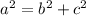
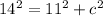
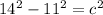
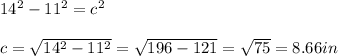
Now, that we already know the the missing side of the rectangle, we can calculate the perimeter using the following formula:

`Perimeter=2base+2length\\\\Perimeter=2*11in+2*8.66in=22in+17.32in=39.32n`

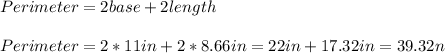
Hence, we have that the perimeter of the rectangle is equal to 39.32".

Have a nice day!