Question

Which polynomial is prime?

X2-36
X2-16
X2-7x + 12
X2-X-20

Answer 1

Explanation:

x^2 - 36 is the difference of two squares and factors as follows:

(x - 6)(x + 6)

x^2 - 16 is the difference of two squares and factors as follows:

(x - 4)(x + 4)

x^2 - 7x + 12 is an easily factored quadratic; the factors are

(x - 3)(x - 4)

x^2 - x - 20 is an easily factored quadratic; the factors are

(x - 5)(x + 4)

I conclude that none of the four expressions is prime.

Answer 2

B.
`x^2+16`

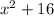
Explanation:

We are asked to find the prime polynomial from our given choices.

We know that a polynomial is prime, when it has only two factors that are 1 and polynomial itself.

Upon looking at our given choices, we can see that each polynomial can be factored except
`x^2+16`.

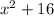We can see that
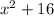`x^2+16` is sum of squares and sum of squares cannot be factored, therefore, polynomial
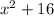`x^2+16` is a prime polynomial.