Question

A city determines that a planned community must have at least 4 acres of developed and open space, and the difference between the number of developed acres, y, and the number of open acres, x, can be no more than 1. Which graph represents the system of inequalities for this scenario? x + y ≤ 4 y – x ≥ 1

Answer 1

Option B.

Explanation:

Let x be the number of open acres and y be the number of developed acres.

It is given that a community must have at least 4 acres of developed and open space.

`x+y\geq 4`

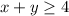
The difference between the number of developed acres, y, and the number of open acres, x, can be no more than 1.

`y-x\leq 1`

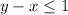
The relative equations of both inequalities are

`x+y=4`

`y-x=1`

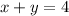
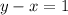
The table of values

For line 1 For line 2

x y x y

0 4 0 1

4 0 -1 0

Plot these ordered pairs on a coordinate plane and draw both lines.

The related line of first inequality is a solid line and shaded area lies above the line because the sign of inequality is ≥.

The related line of second inequality is a solid line and shaded area lies below the line because the sign of inequality is ≤.

Therefore, the correct option is B.

Answer 2

The graph in the attached figure

Explanation:

Let

x ----> the number of open acres

y ----> the number of developed acres

we know that

`x+y \geq 4` -----> inequality A

The solution of the inequality A is the shaded area above the solid line
`x+y=4`

`y-x\leq 1` -----> inequality B

The solution of the inequality B is the shaded area below the solid line
`y-x=1`

so

The graph in the attached figure