Question

Point M is the midpoint of AB if the coordinates of A are (-3,6) and the coordinates of M are (-5,2) what are the coordinates of B ?

Please answer #5

Answer 1

The coordinates of point B are (-7 , -2)

Explanation:

* Lets explain how to solve the problem

- The mid-point (x , y) of the line whose endpoints are (x1 , y1) and

(x2 , y2) is
`x=(x_(1)+x_(2))/(2),y=(y_(1)+y_(2))/(2)`

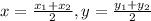
∵ M is the midpoint of AB

∵ The coordinates of point A are (-3 , 6)

∵ The coordinates of point M are (-5 , 2)

- Let the coordinates of point A are (x1 , y1) , The coordinates of

point B are (x2 , y2) and The coordinates of point M are (x , y)

∴ x = -5 , x1 = -3 and y = 2 , y1 = 6

- Lets use the rule of the mid point to find x2 , y2

∵
`-5=(-3+x_(2))/(2)` ⇒ multiply both sides by 2

∴
`-10=-3+x_(2)` ⇒ add 3 to both sides

∴ -7 = x2

∵
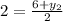`2=(6+y_(2))/(2)` ⇒ multiply both sides by 2

∴
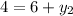`4=6+y_(2)` ⇒ subtract 6 from both sides

∴ -2 = y2

∵ The coordinates of point B are (x2 , y2)

∴ The coordinates of point B are (-7 , -2)