Answer:
c=-5
d=1
Explanation:
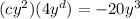
I'm going to reorder the left-hand side. Multiplication is commutative.
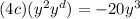
Since the bases are the same in
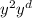 and the operation is multiplication, I'm going to add the exponents giving me:
and the operation is multiplication, I'm going to add the exponents giving me:
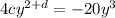
So this implies we have two equations to solve:
 and
and
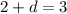
So the first equation can be solved by dividing both sides by 4 giving you
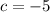 .
.
The second equation can be solved by subtracting 2 on both sides giving you
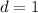 .
.