Answer with explanation:
Number of trophies possessed by me= 7
Number of trophies that is to be selected from 7 trophies =3
⇒⇒So, Chosing 3 out of 7 trophies and arranging them on a shelf requires Concept of Permutation, as order of arrangement is also taken into consideration
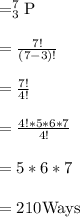
Or
⇒First place can be filled in 7 ways,second place can be filled in 6 ways and third place can be filled in 5 ways.
So total number of ways of selecting 3 trophies from 7 trophies
=7 *6 *5
=210 ways
⇒Now, 3 trophies can be arranged in a shelf in 3! =3 *2*1=6 ways.