Answer: Option a
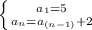
Explanation:
The arithmetic sequences have the following explicit formula
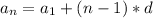
Where d is the common difference between the consecutive terms and
 is the first term of the sequence:
is the first term of the sequence:
The recursive formula for an arithmetic sequence is as follows
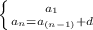
Where d is the common difference between the consecutive terms and
 is the first term of the sequence:
is the first term of the sequence:
In this case we have the explicit formula
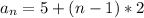
Notice that in this case
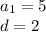
Then the recursive formula is:
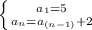
The answer is the option a.