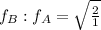Question

Pendulum A has a bob of mass m hung from the string of length L; pendulum B is identical to A except its bob has the length 2L. Compare the frequencies of small oscillations of the two pendulums.

Answer 1

`f_(B): f_(A) = \sqrt{(2)/(1)}`

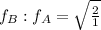
Step-by-step explanation:

For pendulum A: Length = L and gravity = g

The frequency of pendulum A is given by

`f = (1)/(2\pi )\sqrt{(g)/(L)}`

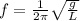
Here, f is the frequency, L be the length

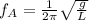`f_(A) = (1)/(2\pi )\sqrt{(g)/(L)}` ... (1)

For pendulum B: Length = 2L, gravity = g

The frequency of pendulum B is given by

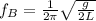`f_(B) = (1)/(2\pi )\sqrt{(g)/(2L)}` .... (2)

Divide equation (1) by (2)

`f_(B): f_(A) = \sqrt{(2)/(1)}`