Answer:
The distance between adjacent antinodes of the standing wave is 1.25 m.
Step-by-step explanation:
Given that,
Frequency f= 120 MHz
We need to calculate the distance between adjacent antinodes of the standing wave
Using formula of distance
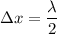 .....(I)
.....(I)
We know that,
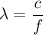
Put the value of
 in to the equation (I)
in to the equation (I)

Where, c = speed of light
f = frequency
Put the all value into the formula
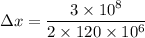
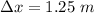
Hence, The distance between adjacent antinodes of the standing wave is 1.25 m.