Question

Verify that the function satisfies the three hypotheses of Rolle's Theorem on the given interval. Then find all numbers c that satisfy the conclusion of Rolle's Theorem. (Enter your answers as a comma-separated list.) f(x) = x3 − x2 − 12x + 7, [0, 4]

Answer 1

Rolle's theorem works for a function
`f(x)` over an interval
`[a,b]` if:

1. 
   `f(x)` is continuous on
   `[a,b]`
2. 
   `f(x)` is differentiable on
   `(a,b)`
3. 
   `f(a)=f(b)`

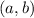
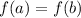
This is our case:
`f(x)` is a polynomial, so it is continuous and differentiable everywhere, and thus in particular it is continuous and differentiable over [0,4].

Also, we have

`f(0)=7=f(4)`

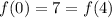
So, we're guaranteed that there exists at least one point
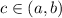`c\in(a,b)` such that
`f'(c)=0`.

Let's compute the derivative:

`f'(x)=3x^2-2x-12`

And we have

`f'(x)=0 \iff x= (1\pm√(37))/(3)`

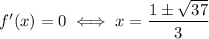
In particular, we have

`(1+√(37))/(3)\approx 2.36`

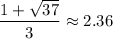
so this is the point that satisfies Rolle's theorem.