Question

If point P is 4/7 of the distance from M to N, what ratio does the point P partition the directed line segment from M to N into?

4:1
4:3
4:7
4:10

Answer 1

Answer: 4:3

Explanation:

Given : A point P is 4/7 of the distance from M to N.

∴ Let the distance between M to N be d.

`\Rightarrow\ MP=(4)/(7)* d=(4d)/(7)`

Also, the point P partition the directed line segment from M to N .

Thus , MN = MP+PN

`\Rightarrow\ d=(4d)/(7)+PN\\\\\Rightarrow\ PN= d-(4d)/(7)=(7d-4d)/(7)\\\\\Rightarrow\ PN=(3)/(7)d`

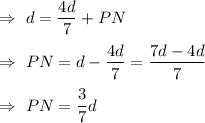
Now, the ration of MP to PN will be :-

`(MP)/(PN)=((4d)/(7))/((3d)/(7))=(4)/(3)`

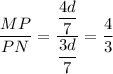
∴ Point P partitioned the line segment MN into 4:3.

Answer 2

4:3

Explanation:

Given that P divides segment MN into 4/7, let MN to be x units in length then

MP = 4/7 x =4x/7 --------(i)

But MN =MP+PN so;

x=4x/7 +PN

x- 4X/7 =PN

3x/7 =PN ----------(ii)

To get the ratio of MP:PN

MP: PN

4x/7:3x/7

MP/PN = 4x/7 / 3x/7

MP/PN =4/3

MP:PN = 4:3