Answer:
95% of students are between 14 and 18 years old
Explanation:
First we calculate the Z-scores
We know the mean and the standard deviation.
The mean is:

The standard deviation is:
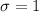
The z-score formula is:
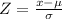
For x=14 the Z-score is:
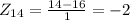
For x=18 the Z-score is:
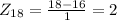
Then we look for the percentage of the data that is between
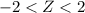 deviations from the mean.
deviations from the mean.
According to the empirical rule 95% of the data is less than 2 standard deviations of the mean. This means that 95% of students are between 14 and 18 years old