Question

Find the length of the segment indicated.

Answer 1

Answer: The length of the indicated segment is 14.45 units.

Step-by-step explanation: We are given to find the length of the indicated segment.

From the figure, we note that

A chord is bisected by the radius of the circle that makes a right-angled triangle with hypotenuse measuring 16.1 units and the other two sides measures x units and 7.1 units.

Using Pythagoras theorem, we get

`x^2+7.1^2=16.1^2\\\\\Rightarrow x^2+50.41=259.21\\\\\Rightarrow x^2=259.21-50.41\\\\\Rightarrow x^2=208.8\\\\\Rightarrow x=\pm√(208.8)\\\\\Rightarrow x=\pm14.45.`

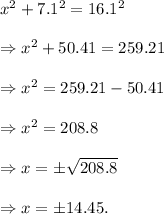
Since x is the length of side of a triangle, so we get

x = 14.45.

Thus, the length of the indicated segment is 14.45 units.