Answer:
* The equation of the median of the trapezoid is 10x + 6y = 39
Explanation:
* Lets explain how to solve the problem
- The slope of the line whose end points are (x1 , y1) , (x2 , y2) is
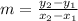
- The mid point of the line whose end point are (x1 , y1) , (x2 , y2) is

- The standard form of the linear equation is Ax + BC = C, where
A , B , C are integers and A , B ≠ 0
- The median of a trapezoid is a segment that joins the midpoints of
the nonparallel sides
- It has two properties:
# It is parallel to both bases
# Its length equals half the sum of the base lengths
* Lets solve the problem
- The trapezoid has vertices R (-1 , 5) , S (! , 8) , T (7 , -2) , U (2 , 0)
- Lets find the slope of the 4 sides two find which of them are the
parallel bases and which of them are the non-parallel bases
# The side RS
∵
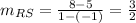
# The side ST
∵
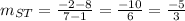
# The side TU
∵
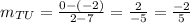
# The side UR
∵
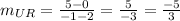
∵ The slope of ST = the slop UR
∴ ST// UR
∴ The parallel bases are ST and UR
∴ The nonparallel sides are RS and TU
- Lets find the midpoint of RS and TU to find the equation of the
median of the trapezoid
∵ The median of a trapezoid is a segment that joins the midpoints of
the nonparallel sides
∵ The midpoint of RS =

∵ The median is parallel to both bases
∴ The slope of the median equal the slopes of the parallel bases = -5/3
∵ The form of the equation of a line is y = mx + c
∴ The equation of the median is y = -5/3 x + c
- To find c substitute x , y in the equation by the coordinates of the
midpoint of RS
∵ The mid point of Rs is (0 , 13/2)
∴ 13/2 = -5/3 (0) + c
∴ 13/2 = c
∴ The equation of the median is y = -5/3 x + 13/2
- Multiply the two sides by 6 to cancel the denominator
∴ The equation of the median is 6y = -10x + 39
- Add 10x to both sides
∴ The equation of the median is 10x + 6y = 39
* The equation of the median of the trapezoid is 10x + 6y = 39