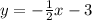Answer:

Explanation:
The slope-intercept form of the equation of a line has the following form:
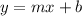
Where m is the slope of the line and b is the intercept with the y axis
In this case we look for the equation of a line that is perpendicular to the line
 .
.
By definition If we have the equation of a line of slope m then the slope of a perpendicular line will have a slope of

In this case the slope of the line
 is
is
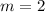 :
:
Then the slope of the line sought is:
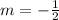
The intercept with the y axis is:
If we know a point
 belonging to the searched line, then the constant b is:
belonging to the searched line, then the constant b is:
 in this case the poin is: (0,-3)
in this case the poin is: (0,-3)
Then:
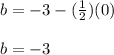
finally the equation of the line is: