Question

Find the equation for the linear function that passes through the points (−5,−6) and (10,3). Answers must use whole numbers and/or fractions, not decimals.

A.Use the line tool below to plot the two points_______

B.State the slope between the points as a reduced fraction________

C.State the y-intercept of the linear function_______

D.State the linear function_________

Answer 1

Slope:
`(3)/(5)`

Y-intercept: -3

Equation:
`y=(3)/(5) x-3`

Graph is attached.

Explanation:

To find your slope using two points, use the slope formula.

`(y2-y1)/(x2-x1) \\`

Your y1 is -6, your y2 is 3.

Your x1 is -5, your x2 is 10.

`(3-(-6))/(10-(-5)) \\\\(9)/(15) \\\\(3)/(5) \\`

Now that you have your slope, use it and one of your points in point-slope form to find your y-intercept.

`y-y1=m(x-x1)\\y-3=(3)/(5) (x-10)\\y-3=(3)/(5) x-6\\y=(3)/(5) x-3`

Answer 2

A. In the graph,

Go 5 units left side from the origin in the x-axis then from that point go downward 6 unit, we will get (-5, -6),

Now, go 10 unit right from the origin in the x-axis then from that point go upward 3 unit, we will get (10, 3),

B. The slope of the line passes through (-5, -6) and (10, 3),

`m=(3-(-6))/(10-(-5))=(3+6)/(10+5)=(9)/(15)=(3)/(5)`

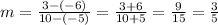
C. Since, the equation of a line passes through
`(x_1, y_1)` with slope m is,

`y-y_1=m(x-x_1)`

Thus, the equation of the line is,

`y+6=(3)/(5)(x+5)----(1)`

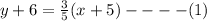
For y-intercept,

x = 0,

`y+6 = (3)/(5)(0+5)\implies y = 3-6=-3`

That is, y-intercept is -3.

D. From equation (1),

`5y + 30 = 3x + 15`

`\implies 3x - 5y = 15`

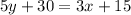
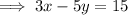
Which is the required linear function.