Answer: The percentage of calcium carbonate is 76.3% and magnesium carbonate is 23.7%.
Step-by-step explanation:
 produced ca be calculated from ideal gas equation:
produced ca be calculated from ideal gas equation:

P= Pressure of the gas = 785 mmHg = 1.03 atm (760 mmHg= 1 atm)
V= Volume of the gas = 1.94 L
T= Temperature of the gas = 25°C=(25+273)K= 298 K (0°C = 273 K)
R= Value of gas constant = 0.0821 Latm\K mol

Mass of
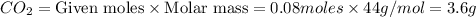
If the mass of calcium carbonate is x g , (7.85-x) g of magnesium carbonate is there.

As, 100 g of
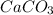 react to give 44 g of
react to give 44 g of
 gas
gas
So, x g of
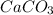 react to give
react to give
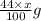 of
of
 gas
gas
The balanced chemical reaction will be:
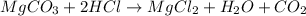
As, 84 g of
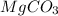 react to give 44 g of
react to give 44 g of
 gas
gas
So,
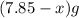 of
of
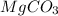 react to give
react to give
 of
of
 gas
gas
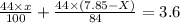
By solving the terms, we get the value of x
x = 5.99 g
The mass of
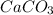 = x = 5.99 g
= x = 5.99 g
The mass of
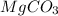 = 7.85 - 5.99 = 1.86 g
= 7.85 - 5.99 = 1.86 g
Now we have to calculate the percentage of magnesium carbonate.

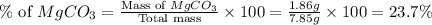
Therefore, the percentage of calcium carbonate is 76.3% and magnesium carbonate is 23.7%.