Answer:
The expected ratio of half-lives for a reaction will be 5:1.
Step-by-step explanation:
Integrated rate law for zero order kinetics is given as:
![k=(1)/(t)([A_o]-[A])](https://img.qammunity.org/2020/formulas/chemistry/college/9hqq5yii0g911kdfij04yhcfhc2gj5gqt0.png)
![[A_o]](https://img.qammunity.org/2020/formulas/chemistry/high-school/38eb24kf04xqy5t88y9g0vzh3m04r4nqgg.png) = initial concentration
= initial concentration
[A]=concentration at time t
k = rate constant
if,
![[A]=(1)/(2)[A_o]](https://img.qammunity.org/2020/formulas/chemistry/college/a8kk0sx6klxe8li1hygs2uhx6qm19gqqbt.png)
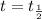 , the equation (1) becomes:
, the equation (1) becomes:
![t_{(1)/(2)}=([A_o])/(2k)](https://img.qammunity.org/2020/formulas/chemistry/college/8iyh1xq8rayabnlvda4gvnp55c0cmvvwom.png)
Half life when concentration was 0.05 M=
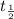
Half life when concentration was 0.01 M=
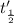
Ratio of half-lives will be:
![\frac{t_{(1)/(2)}}{t_{(1)/(2)}'}=(([0.05 M])/(2k))/(([0.01 M])/(2k))=(5)/(1)](https://img.qammunity.org/2020/formulas/chemistry/college/oq98bf90qas13o3vvgv0kjgm8c3zu63c46.png)
The expected ratio of half-lives for a reaction will be 5:1.