Question

If the fugacity coefficient of the components of a binary mixture are = 0.784, and =0.638 and mole fraction of component 1 is 0.4, write down and expression for the fugacity coefficient Inp, for the mixture.

Answer 1

Answer : The expression for the fugacity coefficient
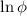`\ln \phi`, for the mixture is, -0.3669.

Explanation : Given,

Fugacity coefficient of component 1 = 0.784

Fugacity coefficient of component 2 = 0.638

Mole fraction of component 1 = 0.4

First we have to calculate the mole fraction of component 2.

As we know that,

`\text{Mole fraction of component 1}+\text{Mole fraction of component 2}=1`

`\text{Mole fraction of component 2}=1-0.4=0.6`

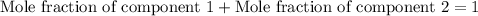
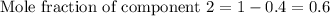
Now we have to calculate the expression for the fugacity coefficient
`\ln \phi`.

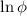Expression used :

`\ln \phi=X_1\ln \phi_1+X_2\ln \phi_2`

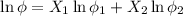
where,

`\phi` = fugacity coefficient

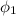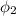`\phi_1` = fugacity coefficient of component 1

`\phi_2` = fugacity coefficient of component 2

`X_1` = mole fraction of of component 1

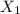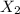`X_2` = mole fraction of of component 2

Now put all the give values in the above expression, we get:

`\ln \phi=0.4* \ln(0.784)+0.6* \ln(0.638)`

`\ln \phi=-0.3669`

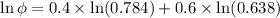
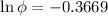
Therefore, the expression for the fugacity coefficient
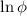`\ln \phi`, for the mixture is, -0.3669.