Question

PLEASE HELP!!

Match each polynomial with the appropriate explanation regarding the roots of the related polynomial equation.

Answer 1

Part 1)
`(x+4)(x-1)(x-2)(x-4)`

The related polynomial equation has a total of four roots, all four roots are real

Part 2)
`(x+1)(x-1)(x+2)^(2)`

The related polynomial equation has a total of four roots, all four roots are real and one root has a multiplicity of 2

Part 3)
`(x+3)(x-4)(x-(2-i))(x+(2-i))`

The related polynomial equation has a total of four roots, two roots are complex and two roots are real

Part 4)
`(x+i)(x-i)(x+2)^(2)`

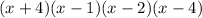
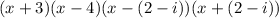
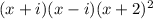
The related polynomial equation has a total of four roots, two roots are complex and one root is real with a a multiplicity of 2

Explanation:

we know that

The Fundamental Theorem of Algebra states that: Any polynomial of degree n has n roots

so

Part 1) we have

`(x+4)(x-1)(x-2)(x-4)`

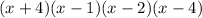
The roots of this polynomial are

x=-4, x=1,x=2,x=4

therefore

The related polynomial equation has a total of four roots, all four roots are real

Part 2) we have

`(x+1)(x-1)(x+2)^(2)`

The roots of this polynomial are

x=-1, x=1,x=-2,x=-2

therefore

The related polynomial equation has a total of four roots, all four roots are real and one root has a multiplicity of 2

Part 3) we have

`(x+3)(x-4)(x-(2-i))(x+(2-i))`

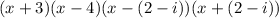
The roots of this polynomial are

x=-3, x=4,x=(2-i),x=-(2-i)

therefore

The related polynomial equation has a total of four roots, two roots are complex and two roots are real

Part 4) we have

`(x+i)(x-i)(x+2)^(2)`

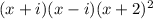
The roots of this polynomial are

x=-i, x=i,x=-2,x=-2

therefore

The related polynomial equation has a total of four roots, two roots are complex and one root is real with a a multiplicity of 2