Question

What is the product?

(x^2-16)/(2x+8) x (x^3-2x^2+x)/(x^2+3x-4)

a. x(x-4)(x-1)/2(x+4)

b. x(x-1)/2

c. (x+4)(x-4)/2x(x-1)

d. (x-4)(x-1)/2x(x+4)

Answer 1

=x(x-4)(x-1)/2(x+4)

Explanation:

=x^2-4^2/2(x+4) * x^3-2x^2+x/x^2+3x-4

=(x+4)(x-4)/2(x+4) * x(x^2-2x+1)/x^2+3x-4

Factor x^2-2x+1 using the perfect square root

=(x+4)(x-4)/2(x+4) * x(x-1)^2/x^2+3x-4

Factor x^2+3x-4 using AC method.

=(x+4)(x-4)/2(x+4) * x(x-1)^2/(x-1)(x+4)

Cancel the common factor of x+4 and x-1

=(x-4)/2(x+4) * x(x-1)/1

=(x-4)x(x-1)/2(x+4)

Reorder the terms

=x(x-4)(x-1)/2(x+4)

Answer 2

Option A is correct.

Explanation:

We need to find the product of

`((x^2-16))/((2x+8)) * ((x^3-2x^2+x))/((x^2+3x-4))`

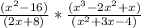
We know (a^2-b^2) = (a+b)(a-b)

so, (x^2-16) = (x)^2-(4)^2 = (x-4)(x+4)

2x+8 Taking 2 common from this term:

2x+8 = 2(x+4)

(x^3-2x^2+x) Taking x common from this term

x(x^2-2x+1) = x(x-1)^2 = x(x-1)(x-1)

(x^2+3x-4) factorizing this term

x^2+4x-x-4 = x(x+4)-1(x+4)

= (x-1)(x+4)

Now, Putting these simplified terms in the given equation:

`((x-4)(x+4))/(2(x+4))*(x(x-1)(x-1))/((x-1)(x+4))`

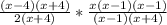
Now cancelling the same terms that are in numerator and denominator

`=((x-4))/(2)*(x(x-1))/((x+4))\\=((x-4)(x)(x-1))/(2(x+4))\\=(x(x-4)(x-1))/(2(x+4))`

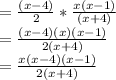
So, Option A is correct.