Question

A rigid sphere is falling from a height of 1m in a fluid of density 1000 m3/kg. The time it took to fall is 500 seconds. If the density of the sphere is 1100 m3/kg and the viscosity of the fluid is 100 cP, what is the diameter of the sphere? (a. 6.1 m , b. 0.61 m , c. 0.061 m , d. 0.0061 m)

Answer 1

0.00191 meter is the diameter of the sphere.

Step-by-step explanation:

Velocity of the rigid sphere =
`v=(1m)/(500 s)`

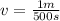
Density of the fluid =
`\rho _f=1000 kg/m^3`

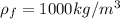
Density of the particle=
`\rho _p=1100 kg/m^3`

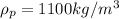
Viscosity of the fluid =
`\eta =100 centiPoise = 1 Poise=0.1 Kg/(m s)`

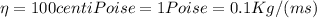
Radius of the sphere = r

`v=(2)/(9)* (\rho _p-\rho _f)/(\eta )gr^2`

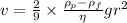
(Terminal velocity)

`(1 m)/(500 s)=(2)/(9)* (1100 kg/m^3-1000 kg/m^3)/(0.1 kg/(m s))* 9.8 m/s^2* r^2`

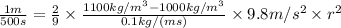
r = 0.00191 m

0.00191 meter is the diameter of the sphere.