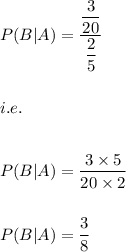Question

An urn contains different colored marbles. The probability of drawing two green marbles from the urn without replacement is 3/20 , and the probability of drawing one green marble is 2/5 .

What is the probability of drawing a second green marble, given that the first marble is green?


3/50

1/2

3/8

1/5

Answer 1

3/50

Explanation:

Answer 2

The probability of drawing a second green marble, given that the first marble is green is:

`(3)/(8)`

Explanation:

Let A denote the event that first marble is green.

B denote the event that the second marble is green.

A∩B denote the event that both the marbles are green.

Let P denote the probability of an event.

We are asked to find:

P(B|A) i.e. probability of drawing a second green marble, given that the first marble is green.

We know that:

`P(B|A)=(P(A\bigcap B))/(P(A))`

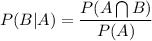
Probability of drawing one green marble is 2/5 i.e.

`P(A)=(2)/(5)`

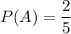
The probability of drawing two green marbles from the urn without replacement is 3/20 i.e.

`P(A\bigcap B)=(3)/(20)`

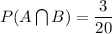
Hence, we have:

`P(B|A)=((3)/(20))/((2)/(5))\\\\\\i.e.\\\\\\P(B|A)=(3* 5)/(20* 2)\\\\\\P(B|A)=(3)/(8)`