Question

I have nooooo clue, please help

Answer 1

case a)
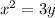`x^(2)=3y` ----> open up

case b)
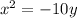`x^(2)=-10y` ----> open down

case c)
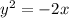`y^(2)=-2x` ----> open left

case d)
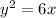`y^(2)=6x` ----> open right

Explanation:

we know that

1) The general equation of a vertical parabola is equal to

`y=a(x-h)^(2)+k`

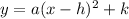
where

a is a coefficient

(h,k) is the vertex

If a>0 ----> the parabola open upward and the vertex is a minimum

If a<0 ----> the parabola open downward and the vertex is a maximum

2) The general equation of a horizontal parabola is equal to

`x=a(y-k)^(2)+h`

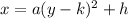
where

a is a coefficient

(h,k) is the vertex

If a>0 ----> the parabola open to the right

If a<0 ----> the parabola open to the left

Verify each case

case a) we have

`x^(2)=3y`

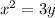
so

`y=(1/3)x^(2)`

`a=(1/3)`

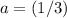
so

`a>0`

therefore

The parabola open up

case b) we have

`x^(2)=-10y`

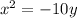
so

`y=-(1/10)x^(2)`

`a=-(1/10)`

`a<0`

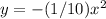
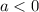
therefore

The parabola open down

case c) we have

`y^(2)=-2x`

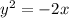
so

`x=-(1/2)y^(2)`

`a=-(1/2)`

`a<0`

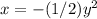
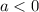
therefore

The parabola open to the left

case d) we have

`y^(2)=6x`

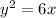
so

`x=(1/6)y^(2)`

`a=(1/6)`

`a>0`

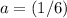
therefore

The parabola open to the right