Answer:
The coefficient of friction is 0.34
Step-by-step explanation:
It is given that,
Mass of the load, m = 1000 kg
It is dragged up an incline at 10 degrees to the horizontal by a force of 5 KN applied in the most effective direction, F = 5 × 10³ N
We need to find the coefficient of friction between the surface and the load. From the attached figure, the load is dragged up with a force of F. A frictional force f will also act in this scenario.
So,
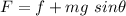
Since,
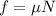
or
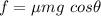
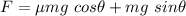
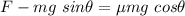
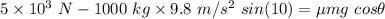
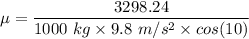
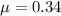
So, the coefficient of friction is 0.34. Hence, this is the required solution.