Question

For the function f(x)=
`(√(x) )/(5) +2`

A. ƒ –1(x) = 25(x – 2)2, x ≥ 2

B. ƒ –1(x) = 25x2 – 2, x ≥ 0

C. ƒ –1(x) = 5(x – 2)2, x ≥ 0

D. ƒ –1(x) = 25(x – 2)2, x ≥ 0

Answer 1

`f^(-1)(x)=25(x-2)^2`

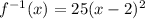
Explanation:

The inverse function can be found by solving ...

f(y) = x

(√y)/5 +2 = x

(√y)/5 = x -2 . . . . . subtract 2

√y = 5(x -2) . . . . . . multiply by 5

y = 25(x -2)² . . . . . square both sides

The inverse function is ...

`f^(-1)(x)=25(x-2)^2`

_____

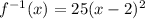
About the graph

The inverse of a function is its mirror image across the line y = x.