Question

5. A deck should have a perimeter of 90 feet and a minimum area

of 450 square feet. Write and solve an inequality to find the
possible width of the deck.​

Answer 1

The minimum value of the width is 15 ft and the maximum value of the width is 30 ft

Explanation:

Let

x -----> the length of the deck

y ----> the width of the deck

we know that

The perimeter of the deck is equal to

`P=2(x+y)`

`P=90\ ft`

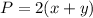
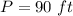
so

`90=2(x+y)`

`45=(x+y)`

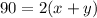
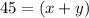
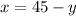`x=45-y` -----> equation A

The area is equal to

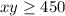`xy\geq 450` -----> inequality B

`(45-y)y\geq 450\\ \\-y^(2) +45y-450\geq 0`

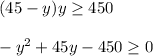
Solve the quadratic equation by graphing

The possible width of the deck are the values of y in the interval

[15,30]

All real numbers greater than or equal to 15 ft and less than or equal to 30 ft

see the attached figure

The minimum value of the width is 15 ft and the maximum value of the width is 30 ft