ANSWER
![\sqrt[3]{- 729{a}^(9) {b}^(6) } = - 9 {a}^(3) {b}^(2)](https://img.qammunity.org/2020/formulas/mathematics/middle-school/9dpmjjr4cz3w1lys8syhybs2u79lmh5mvi.png)
EXPLANATION
We want to find the cube root of
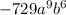
We express this symbolically as:
![\sqrt[3]{- 729 {a}^(9) {b}^(6) }](https://img.qammunity.org/2020/formulas/mathematics/middle-school/vc4o38ibu44gfhjr7a3s4n1cwi0g876rbp.png)
The expression under the radical called the radicand.
We need to express this radical in exponential form using the property,
![{x}^{ (m)/(n) } = \sqrt[n]{ {x}^(m) }](https://img.qammunity.org/2020/formulas/mathematics/middle-school/9kncfnovx038h83vv4wyqp5pk8icd4zyue.png)
Applying this rule gives us:
![\sqrt[3]{- 729 {a}^(9) {b}^(6) } = ({- 729 {a}^(9) {b}^(6)})^{ (1)/(3) }](https://img.qammunity.org/2020/formulas/mathematics/middle-school/ex1ybn81dyvnagfjz9n4l5v5qrqer5etq1.png)
![\sqrt[3]{- 729{a}^(9) {b}^(6) } = ({- {9}^(3) {a}^(9) {b}^(6)})^{ (1)/(3) }](https://img.qammunity.org/2020/formulas/mathematics/middle-school/30aytrxgrbveqzw0x5t098cic5hcvdujn0.png)
Recall that
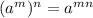
We apply this rule on the RHS to get,
![\sqrt[3]{- 729{a}^(9) {b}^(6) } = ({- {9}^{3 * { (1)/(3) } } {a}^{9 * { (1)/(3) } } {b}^{6 * { (1)/(3) } }})](https://img.qammunity.org/2020/formulas/mathematics/middle-school/qmcagqn1lyert6cro0ud4z8p8y6rrv2m64.png)
This simplifies to
![\sqrt[3]{- 729{a}^(9) {b}^(6) } = - 9 {a}^(3) {b}^(2)](https://img.qammunity.org/2020/formulas/mathematics/middle-school/9dpmjjr4cz3w1lys8syhybs2u79lmh5mvi.png)