Question

If you deposit $14,000 in an account that pays 7.47% annual interest compounded continuously. Remember A=Pe^rt.

What is the balance after 1 year? 5 years? 10 years? 25 years?

Answer 1

Answer: After 1 year = $15085.85168

After 5 years = $20339.34789

After 10 years = $29549.21947

After 25 years = $90609.34284

Explanation:

14000e^.0747 (however many years)

Answer 2

The balance after 1 year, 5 years, 10 years, 25 years are 15085.85, 20339.35, 29549.22 and 90609.34 respectively.

Explanation:

It is given that the principle amount is $14,000 and interest rate is 7.47%.

The formula for amount is

`A=Pe^(rt)`

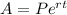
Where, P is principle, r is rate of interest and t is time in years.

Substitute P=14000 and r=0.0747 in the above equation.

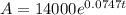`A=14000e^(0.0747t)` ..... (1)

Substitute t=1 in equation (1) to find the balance after 1 year.

`A=14000e^(0.0747(1))=15085.851678\approx 15085.85`

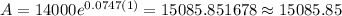
Substitute t=5 in equation (1) to find the balance after 5 year.

`A=14000e^(0.0747(5))=20339.3478896\approx 20339.35`

Substitute t=10 in equation (1) to find the balance after 10 year.

`A=14000e^(0.0747(10))=29549.2194696\approx 29549.22`

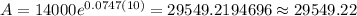
Substitute t=25 in equation (1) to find the balance after 25 year.

`A=14000e^(0.0747(25))=90609.3428426\approx 90609.34`

Therefore the balance after 1 year, 5 years, 10 years, 25 years are 15085.85, 20339.35, 29549.22 and 90609.34 respectively.